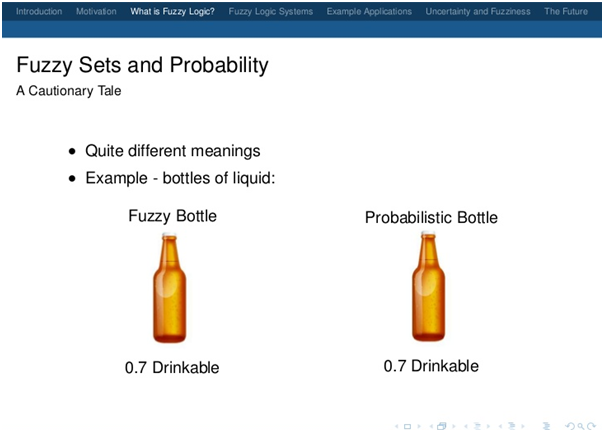
The two are very closely related. As we’ll see when we start looking at fuzzy logic, the basic connectives in fuzzy judgment are definite in about the same way as the parallel operation in probability theory.
The key difference is meaning.
There are two major schools of considerations in probability theory, and they all allocate a very dissimilar meaning to probability. I’m disappearing to greatly simplify, but the two schools are the frequent and the Bayesians.
First, present are the frequentists. To the frequentists, possibility is defined by trial. If you say that an occasion E has a chance of, say, 60%, what that means to the frequentists is that if you could replicate an research observe the happening or non-occurrence of E an endless number of times, then 60% of the time, E would have occur. That, in spin, is in use to indicate that the incident E has an intrinsic probability of 60%.
In fuzzy logic, we’re annoying to imprison the necessary possessions of indistinctness. If I say that a human whose height is 2.5 meters giant, that’s a accurate declaration. If I say that one more individual whose tallness is only 2 meters is tall, that’s still true – but it’s not as right as it be for the one 2.5 meter tall. I’m not axiom that in a repeatable testing, the first person would be tall more often than the moment.
And, in the other path: fuzzy sense isn’t largely functional for argument regarding fraction details. If you allowable second-order reason, you might have fuzzy meta-predicates that describe your faith about hard first-order predicates.
So probability theory doesn’t imprison the necessary property of sense (partial truth) which is the aim of fuzzy logic – and fuzzy logic doesn’t arrest the essential goods of connotation (partial knowledge) which is the goal of chance premise.